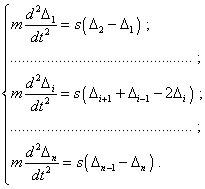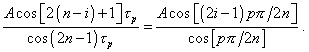Volume 4 (1999), No 4, pp. 5-13

9

Solutions for finite elastic lumped lines

Notice one more interesting property of the yielded solutions. If in a finite line in Fig. 2 we retain only one element, i.e. n = 1, then i = 1 and the solution of (13) will be transformed to the form

It means, when reducing the number of line elements to 1, the solution automatically transformed to the expression describing the motion of a solitary element without constraints. Further we will multiply see the property of solutions to transform from more complicated models to those simple, which is an important advantage of the exact solutions.

3.2. Free vibrations of a line with unfixed start and end

As we know, the following homogeneous system of differential equations without external force F(t) corresponds to the elastic line free vibrations:

In distinction from forced vibrations, in such system the vibrations can arise not in the whole range of frequencies but only in its definite part, and their spectrum will be discrete. For the investigated unfixed-ends model, the conditions of vibrations are determined by the expression

where

Proceeding from (30), the natural (allowed) vibration frequencies are determined by the expression

In accordance with the allowed frequencies (31), the solution of (29) has the form

As we see, the structure of expression (32) for the amplitude basically differs from such in (6). In (32) the amplitude is presented by the ratio of trigonometric functions with a complex system of angular shifts, while (6) being the basis of the conventional solution had the form of a trivial trigonometric function. Such structure is typical for all lines where the reflection processes take place, even in a semi-finite line presented in [1], because it is just the result of account of the process of multiple reflections from the boundaries. Due to this, the vibration amplitude becomes dependent on the line length and on the number of an allowed mode, not only on the element number as in (6); the denominator of (32) determines it.

To determine the pattern of this dependence, conveniently transform the terms of (32) characterising the vibration amplitude, noting (30). We will yield

The expression (33) shows that with the increasing number of the mode, the denominator monotonously decreases but does not vanish, since

Due to this, the amplitude grows monotonously but does not reach the infinite value at the maximally possible mode. With the growing number of elements, this amplitude maximum increases, too and turns into infinity only when passing to a distributed line.

Thus, we see that with free vibrations, only finite vibrations can arise in lumped lines, though the values of natural frequencies coincide with the resonance conditions (14) for forced vibrations. Practically it means the known fact that if some solitary pulse has acted upon a finite system, then finite vibrations arise in it, and if this pulse repeats with resonance frequency, it will lead to the line destruction.

Besides, as it follows from the carried out analysis, if in the regime of free vibrations the amplitude monotonously changed with the mode number, then with the forced vibrations in the band of periodical regime, the amplitude can multiply reach the maximum and minimum dependently on the length and parameters of a line. This difference has the following reason. The forced vibration spectrum has a continuous pattern, while in free vibrations the line spectrum is inherent; this causes the monotonous discrete sampling standing from the continuous periodical regularity.

In the light of revealed essential distinctions between the vibration types, we should note that the solution (32) is, nevertheless, similar to (13) in its block structure.