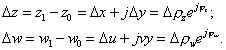104

S.B. Karavashkin and O.N. Karavashkina

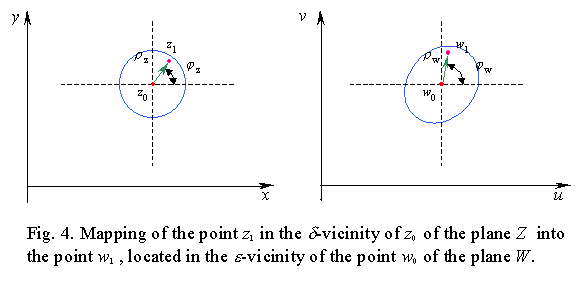
The possibility itself to generalise the definition of differentiability of functions of complex variable, with remaining the analyticity definition, means not only broadening the domain in which the definition 1 is true, but reflects the essential transformation of concept of the differential and derivative of a complex function. To explain, consider some point  z₁ in the 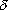 -vicinity of the point  z₀ and its mapping into the point  w₁ located in the  -vicinity of the point  w₀ (see Fig. 4). Basing on this construction, we can write as follows:

Then

The expression (13) shows that when mapping in the complex plane, the derivative does not define the tangent of inclination angle of the tangent line at the studied point, as it was in case of real variable, but characterises the geometrical transformation of a path with the mapping. And this naturally must reflect in the formulation of definitions.

At the same time, we can easy trace the reason, why the limits in the definition 2 must be equal. This definition was constructed by analogy with the differentiability condition of functions of one real variable, where the requirement, the limits to be equal, was formulated in the following way:

Definition 3. "The number b is the limit value of the function y = f (x) at the point x = a (or the limit of a function at x a), if for any sequence x₁,  x₂, ...,  x_n, ... of argument x converging to a, whose elements  z_n distinguish from a  (x_n 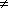a), the corresponding sequence f(x₁),  f(x₂), ...,  f(x_n), .. of the function values converged to b" [5, p.98, with our italicisation - authors]. Shilov confirms: "This definition ((2) - authors) of a derivative of a complex-variable function is by its form alike the definition of a real-function derivative in a real domain. The definition of a derivative of a real-variable function is a particular case of this presented… However with the external similarity between the derivatives in real and complex domains, there is a number of essential distinctions" [6, p.397].

For real-variable functions this severity of definition 3 is quite justified due to the fact that in this case by the concept of any converging sequence one means some countable set    x₁,  x₂, ...,  x_n, ... mapped onto the supposed smooth curve in the -vicinity of a point b = f(a). Due to it one can say that the set S constituted of the elements of a sequence x₁,  x₂, ...,  x_n, ... is everywhere dense and nested for the countable set C of all sequences converging at the point a.

In case of complex functions we deal with 2D mapping of the domain Z onto 2D domain of values W. With it each arc in the -vicinity of the point z₀  has its only (in case of 1D mapping) image in the -vicinity of the point  w₀, and the - and -vicinities themselves become 2D. Hence, the set  S constituted of elements of the sequence z₁,  z₂, ...,  z_n, ... , though it remains nested into the set of sequences C   converging to  z₀, stops be everywhere dense in the set   C , as it is constituted only of elements belonging to one arc of a set of arcs crossing at the point  z₀. This feature requires to take into account both the convergence of all sequences along one direction and all directions of contraction. However this last does not effect on the substantiation of the limit itself for each sequence, since they all converge to the common point  z₀, and their images - to  w₀. But the contraction velocities in different directions can be generally different.