| SELF |
4 |
| S.B.
Karavashkin and O.N. Karavashkina |

|
To make the analysis of (11) easier and more visual, plot
the space-time diagram of the vector  (x, t) amplitude variation,
beginning from some arbitrary chosen initial time t0 , as we did it in
[10]. The general form of this diagram is shown in the centre of Fig. 2. As is usual for delaying processes, the
phase of vector (x, t) amplitude variation,
beginning from some arbitrary chosen initial time t0 , as we did it in
[10]. The general form of this diagram is shown in the centre of Fig. 2. As is usual for delaying processes, the
phase of vector  (x, t) oscillations displaces with growing
x . Different parts of the wave appear in the domain of paths picked out being
well-visible due to the dotted lines dropped from the sides of paths to the diagram.
Therefore at the opposite sides of paths there are indicated the different values of the
momentary amplitude of the vector (x, t) oscillations displaces with growing
x . Different parts of the wave appear in the domain of paths picked out being
well-visible due to the dotted lines dropped from the sides of paths to the diagram.
Therefore at the opposite sides of paths there are indicated the different values of the
momentary amplitude of the vector  (x, t) . It evidences that the circulation
of the vector (x, t) . It evidences that the circulation
of the vector  (x,
t) is non-identical to zero. We can easy calculate this circulation of the
vector, noting that the wave front is plane and the flux is 1D. Due to it, the integration
in the expression (11) reduces to a summation of scalar products of the momentary values
of vector (x,
t) is non-identical to zero. We can easy calculate this circulation of the
vector, noting that the wave front is plane and the flux is 1D. Due to it, the integration
in the expression (11) reduces to a summation of scalar products of the momentary values
of vector  (x,
t) amplitude by the length l of corresponding sides of the chosen path. (x,
t) amplitude by the length l of corresponding sides of the chosen path. The results yielded for each of
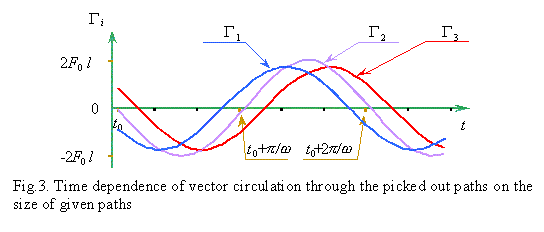
chosen paths at the instances shown in Fig. 2 (left) are shown in Fig. 2 (right).
The calculations show that,
actually, the circulation  i is not identically zero. Its
value varies in time and does not coincide for all three picked out paths. It is graphical
in the plot i is not identically zero. Its
value varies in time and does not coincide for all three picked out paths. It is graphical
in the plot  i(t)
shown in Fig. 3. We see that both the amplitude and phase of oscillations of the
circulation of vector in time depend on the paths size. i(t)
shown in Fig. 3. We see that both the amplitude and phase of oscillations of the
circulation of vector in time depend on the paths size. |
|
We can write it as follows: |
 |
(12) |
where  xi = xi - x0
; i = 1, 2, 3. xi = xi - x0
; i = 1, 2, 3.
As  i ( i (  xi) appeared because
of finite velocity of a wave space-propagation, we can express xi) appeared because
of finite velocity of a wave space-propagation, we can express  xi
through the time delay characteristic of wave: xi
through the time delay characteristic of wave: |
 |
(13) |
where  ti is the delay time, with the
wave passing between the opposite sides of the ith path picked out. ti is the delay time, with the
wave passing between the opposite sides of the ith path picked out.
Substituting (13) into (12), we yield |
 |
(14) |
Noting that the square size of the path picked out depends
on  xi
too as xi
too as |
 |
(15) |
| the specific
circulation is equal to |
 |
(16) |
It means, the curl of vector also will not vanish: |
 |
(17) |
We see from (17) that the value of
curl of vector  (x,
t) in case of dynamical field depends on the frequency of field variation and on
the wave propagation velocity, and the phase of curl variation leads the phase of vector
oscillations by (x,
t) in case of dynamical field depends on the frequency of field variation and on
the wave propagation velocity, and the phase of curl variation leads the phase of vector
oscillations by  /2 . When passing to the stationary fields, i.e. at /2 . When passing to the stationary fields, i.e. at 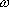  0 and/or c 0 and/or c   , the right part of expression (17)
automatically vanishes, agreeing with the conventional concept (2). , the right part of expression (17)
automatically vanishes, agreeing with the conventional concept (2).
With all simplicity of this
derivation and obvious result, we note that when transiting to the stationary field, the
curl of vector vanishes. It can be easy generalised for the case of arbitrary flux of
vector. |


