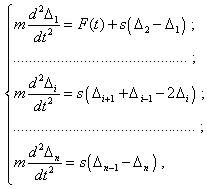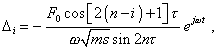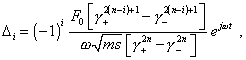Volume 4 (1999), No 4, pp. 5-13

7

Solutions for finite elastic lumped lines

3.1. Forced vibrations of a line with unfixed start and end

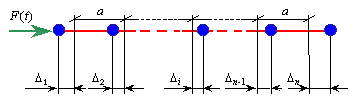
In Fig. 2 we show the mechanical model of a finite line with unfixed start and end.

Fig. 2. Mechanical model of a finite lumped line with unfixed start and end

The following system of differential equations corresponds to this model:

where, the same as in [1], _i is the momentary shift of the ith element of line, F (t) is the external force affecting the line, F (t) = F₀ e and F₀   is the amplitude of the external force.

The same as for infinite lines, (12) has in general case three basically different solutions depending on the ratio of to 1, where

s is the stiffness coefficient of the line.

3.1.1 For finite lines the periodic solution related to < 1 is

where

.

This solution describes standing waves, which is determined by the constant phase delay of vibrations for all line elements. But unlike the conventional solutions (6), the exact solution structure has basically other form. With

an infinite resonance will take place in the line. But in other cases, the vibration amplitude is finite and determined by the value sin 2n . With

it will be minimal but not zero, as we used to think for resonant lines. The typical vibration pattern is shown in Fig. 3. But the main that follows from the yielded solutions is that line vibrations are not limited by the critical frequency.

Fig. 3. The typical appearance of vibrations for finite lumped lines in the periodic regime (m = 0,01 kg; s = 100 N/m; a = 0,01 m; n = 8; F₀ = 0,6 N;  f = 15 Hz)

3.1.2 In the aperiodical regime corresponding to  >1 the exact solution takes the form

where

Just as in case of infinite lines in [1], we can see the antiphase vibrations, but damping not so fast as in infinite lines. In infinite lines the damping distributes along the whole line. In [1], when analysing free vibrations, we mentioned the resemblance in vibrations for finite and infinite lines and that in the aperiodical case the damping begins from the point of energy input into a vibrant system. In Fig. 4 we can see namely this phenomenon, when the damping passes from the first to the last element, distributing uniformly along the whole line, which confirms the analogy indicated in [1].

3.1.3. For the critical regime corresponding to = 1, the changes are also interesting:

In a finite line these vibrations have a damping pattern, not remaining their amplitude, as in infinite lines. But unlike the aperiodical regime, the damping has not a power-type but linear pattern. We can see these features in Fig. 4.

Fig. 4. Damping in the critical regime (f = f_crit = 31,8 Hz. The values of rest parameters are same as in Fig. 3)